Band gap of strained AlGaInP on GaAs substrate

Input Files:

• AlGaInP_on_GaAs_1D_nnp.in

Scope:

In this tutorial we want to study the band gaps of strained \(Al_xGa_yIn_{1-x-y}P\) on a \(GaAs\) substrate. The material parameters are taken from [VurgaftmanJAP2001].

Output Files:

• strain\strain_simualtion.dat

• strain\hydrostatic_strain.dat

• bias_00000\bandedges.dat

Strain

To understand the effect of strain on the band gap on the individual components of the quaternary \(Al_xGa_yIn_{1-x-y}P\), we first examine the effects on

1. \(AlP\) strained tensely with respect to \(GaAs\)

2. \(GaP\) strained tensely with respect to \(GaAs\)

3. \(InP\) strained compressively with respect to \(GaAs\)

4. \(Al_xGa_{1-x}P\) strained tensely with respect to \(GaAs\)

5. \(Ga_xIn_{1-x}P\) strained with respect to \(GaAs\)

6. \(Al_xIn_{1-x}P\) strained with respect to \(GaAs\)

7. \(Al_{0.4}Ga_{0.6}P\) strained tensely with respect to \(GaAs\)

8. \(Ga_{0.4}In_{0.6}P\) strained compressively with respect to \(GaAs\)

9. \(Al_{0.4}In_{0.6}P\) strained compressively with respect to \(GaAs\)

Each material layer has a length of 10 nm in the simulation. The material layers 4), 5) and 6) vary their alloy contents linearly, i.e.

4. \(Al_xGa_{1-x}P\): x = 0.0 to x = 1.0 (from 10 nm to 20 nm)

5. \(Ga_xIn_{1-x}P\): x = 0.0 to x = 1.0 (from 30 nm to 40 nm)

6. \(Al_xIn_{1-x}P\): x = 1.0 to x = 0.0 (from 50 nm to 60 nm)

There is no external stress applied to the structure, so Poisson’s ratio holds. All layers are strained pseudomorphically with respect to a \(GaAs\) substrate (i.e. the layers are biaxially strained in the plane perpendicular to the growth direction to match the lattice constant of \(GaAs\)).

The biaxial strain in the layers can be calculated with this formula:

\[e_{yy} = e_{zz} = \frac{a_\mathrm{substrate} - a}{a}\]

where \(a\) is the lattice constant. The output of the strain tensor can be found in this file: strain\strain_simualtion.dat

The hydrostatic strain is the trace of the strain tensor and corresponds to the volume deformation:

\[e_\mathrm{hydro} = Tr(e_{ij}) = e_{xx} + e_{yy} + e_{zz}\]

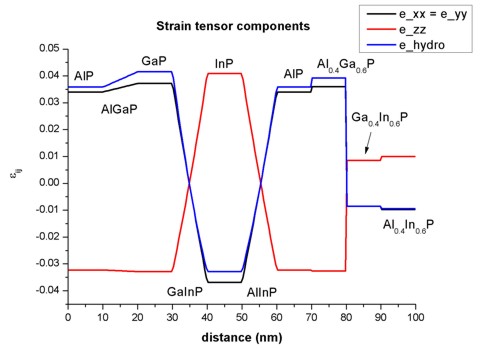
Figure 2.5.2.37 Strain tensor components

band gaps

Figure 2.5.2.38 shows the conduction band edges at the Gamma, L and X points and the heavy hole, light hole and split-off hole valence bands. The red line shows that band gap, i.e. the difference between the lowest conduction band minimum and the valence band maximum. The band gap maximum occurs at \(Al_{0.55}In_{0.45}P\) (2.355 eV).

The conduction and valence band edges have been obtained taking into account the shifts and splittings of the bands due to strain and deformation potentials.

Note that conduction and valence band offsets are not taken into account in this plot. The zero of energy was taken to be the unstrained heavy hole / light hole band edge.

Due to strain, the degeneracy of the heavy and light hole is lifted. Also, the X band splits into two X bands (2-fold and 4-fold degeneracy).

In the case of tensile (compressive) strain, the light (heavy) hole band is the valence band maximum.

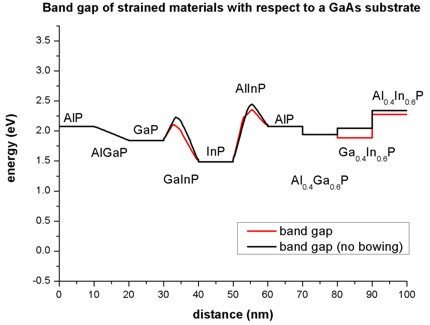
Figure 2.5.2.38 Band edge and band gap profile

Note that the material parameters include band gap bowing.

Figure 2.5.2.39 compares the overall band gap to the case where band gap bowing has been neglected.

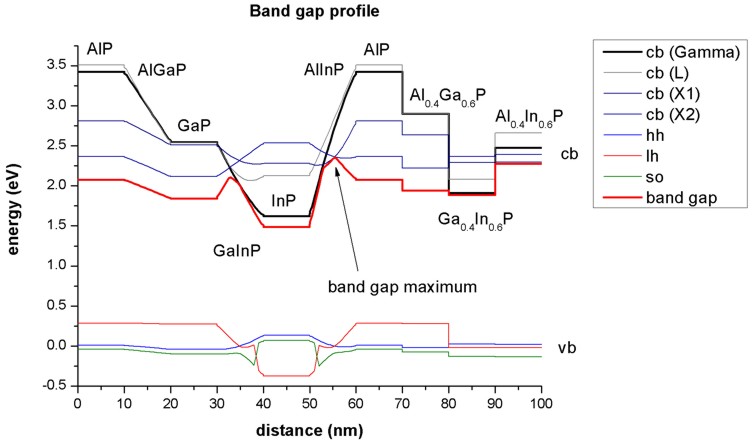
Figure 2.5.2.39 Ban dgap profile

The nextnano++ software supports quaternaries in comparison with nextnano³:

quaternary_constant{
    name = "Al(x)Ga(y)In(1-x-y)P"
    alloy_x = 0.255
    alloy_y = 0.255
}

Appendix E of the PhD thesis of T. Zibold ([ZiboldPhD2007]) is related to the nextnano++ implementation of quaternaries.

Last update: nn/nn/nnnn