Interband absorption of a GaAs cylindrical quantum wire

Section author: Naoki Mitsui (simulation), Brandon Loke (write-up and visualisation)

This tutorial calculates the optical spectrum of a GaAs cylindrical quantum wire with infinite barriers. The formulas used to calculate absorption spectra will be highlighted and a brief explanation of the output files will be given.

For the detailed scheme of the calculation of the optical matrix elements or absorption spectrum, please see our 1D optics tutorial: Optical absorption for interband and intersubband transitions For the corresponding tutorial for the intraband absorption, please see Intersubband absorption of a GaAs cylindrical quantum wire Input file:

• 2Dcircular_infinite_wire_GaAs_inter_nnp.in

Note

Figures in this tutorial will be generated with nextnanopy.

The corresponding Jupyter Notebook used to generate the figures in this tutorial can be found here at 2DInterbandQuantumCylinder.ipynb.

Structure

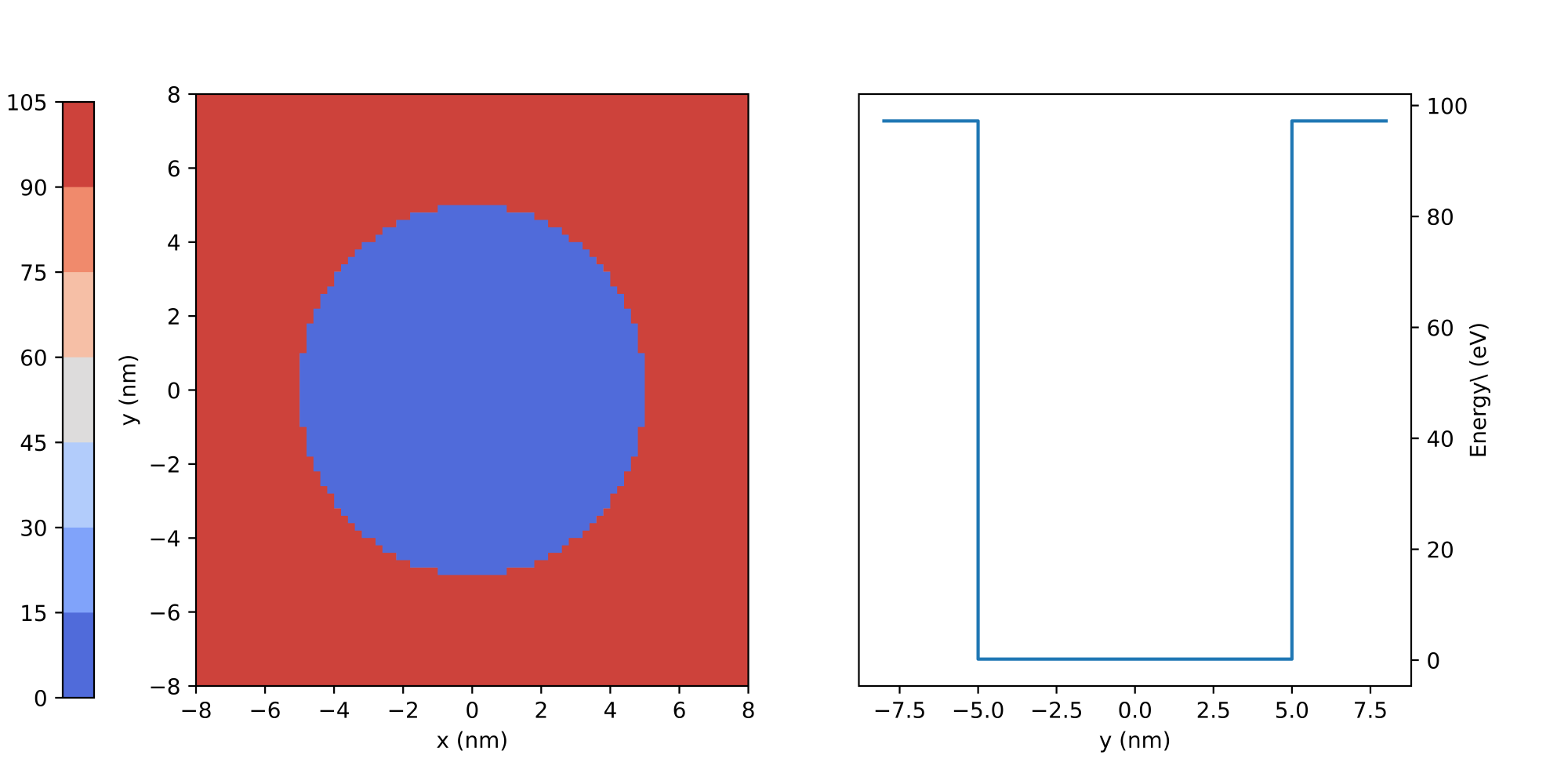
Figure 2.5.12.57 Left: Conduction band edge for cylindrical quantum wire. Right: Slice of the band edge along \(x=0\).

The above figures show the Gamma band edge of the circular GaAs region and the barrier region. We model the infinite barrier by assigning 100 eV for the band edge of AlAs barrier region from database{} section. Please see the input file for the details.

The parameters used in this simulation are as follows.

Property	Symbol	Value [unit]
quantum wire radius	\(R\)	5 [nm]
barrier height	\(E_b\)	92 [eV]
effective electron mass	\(m_e\)	0.0665
refractive index	\(n_r\)	3.3
doping concentation (n-type)	\(N_D\)	5\(\cdot\)1018 [cm-3]
linewidth (FWHM)	\(\Gamma\)	0.01 [eV]
temperature	\(T\)	300 [K]

The run{} section is specified as follows:

run{
    poisson{}
    quantum{}
    optics{}
}

Then the simulation follows these steps:

1. Poisson equation is solved with the setting specified in the poisson{} (optional) section.

2. “Schrödinger” equation is solved with the setting specified in the quantum{ } section.

3. “Schrödinger” equation is solved again with the setting specified in the optics{ } section and optical properties are calculated.

Note

• If quantum_poisson{} is specified instead of quantum{}, Poisson and Schrödinger equations are solved self-consistently.

• optics{} requires that kp8 model is used in the quantum region specified in quantum{}.

• In this tutorial the kp parameters are adjusted so that the conduction and valence bands are decoupled from each other. Thus the single-band Schrödinger equations are solved effectively by the kp solver.

Spectra of optical absorption accompanied by the excitation of charge carriers (state \(n\to m\)) in condensed matter is calculated on the basis of Fermi’s golden rule [ChuangOpto1995] in the dimenstion of (length)^-1:

(2.5.12.5)\[\alpha(\vec{\epsilon},\omega)=\frac{\pi e^2}{n_sc\varepsilon_0 m_0^2\omega}\frac{1}{V}\sum_{n>m}\sum_{\text{k}_z} |\vec{\epsilon}\cdot\vec{\pi}_{nm}(\text{k}_z)|^2 (f_m(\text{k}_z)-f_n(\text{k}_z))\mathcal{L}(E_n(\text{k}_z)-E_m(\text{k}_z)-\hbar\omega),\]

where

• \(\text{k}_z\) is the Bloch wave vector along translation-invariant directions. In 2D simulation this is 1D vector.

• \(E_{n}(\text{k}_z)\) is the energy of eigenstate \(n\). The first sum runs over the pair of states where \(E_{n}(\text{k}_z)>E_{m}(\text{k}_z)\).

• \(f_n(\text{k}_z)\) is the occupation of eigenstate \(n\).

• \(\vec{\epsilon}\) is the optical polarization vector defined in optics{ region{} }.

• \(\vec{\pi}=\vec{p}+\frac{1}{4m_0c^2}(\sigma\times\nabla V)\) where \(\vec{p}\) is the canonical momentum operator and \(\frac{1}{4m_0c^2}(\sigma\times\nabla V)\) is the contribution of spin-orbit interaction.

• \(\vec{\pi}_{nm}(\text{k}_z)=\langle n|\vec{\pi}|m\rangle\).

• \(\vec{\epsilon}\cdot\vec{\pi}_{nm}(\text{k}_z)\) is known as the optical matrix elements.

• \(\mathcal{L}(E_n(\text{k}_z)-E_m(\text{k}_z)-\hbar\omega)\) is the energy broadening function.

  • When energy_broadening_lorentzian is specified in optics{ region{} },

    \(\mathcal{L}(E_n-E_m-\hbar\omega)=\frac{1}{\pi}\frac{\Gamma/2}{(E_n-E_m-\hbar\omega)+(\Gamma/2)^2}\)

    where \(\Gamma\) is the FWHM defined by energy_broadening_lorentzian.

  • When energy_broadening_gaussian is specified in optics{ region{} },

    \(\mathcal{L}(E_n-E_m-\hbar\omega)=\frac{1}{\sqrt{2\pi}\sigma}\exp{\big(-\frac{(E_n-E_m-\hbar\omega)^2}{2\sigma^2}\big)}\)

    where energy_broadening_lorentzian defines the FWMH \(\Gamma=2\sqrt{\ln 2}\cdot\sigma\)

  • When neither energy_broadening_lorentzian nor energy_broadening_gaussian is specified in optics{ region{} }, \(\mathcal{L}\) is replace by the delta function \(\delta(E_n-E_m-\hbar\omega)\).

  • It is also possible to include both Lorentzian and Gaussian broadening (Voigt profile).

The detailed calculation scheme of the optical matrix elements \(\vec{\epsilon}\cdot\vec{\pi}_{nm}(\text{k}_z)\) and the absorption spectrum \(\alpha\) is described in Optical absorption for interband and intersubband transitions.

Results

Absorption

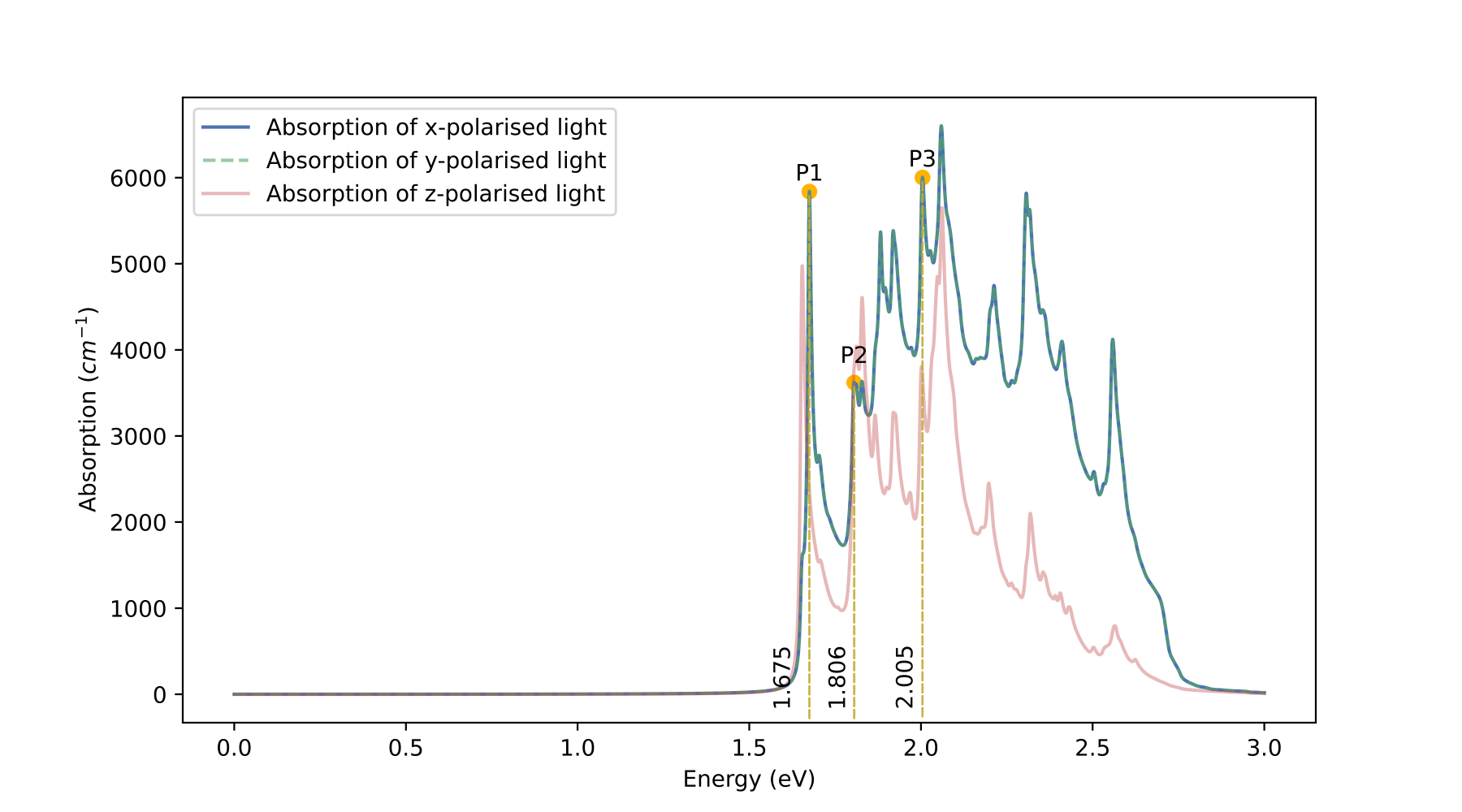
Figure 2.5.12.58 Calculated absorption spectrum \(\alpha(\vec{\epsilon}, E)\) for \(\vec{\epsilon}=\hat{x},\hat{y},\hat{z}\).

Figure 2.5.12.58 shows the calculated \(\alpha(\vec{\epsilon}, E)\) specified in \Optics\absorption_~.dat for each polarization x, y, and z. The absorptions for the x- and y-polarisation are identical due to the rotational symmetry of the quantum cylinder in the x-y plane. It is observed that there are peaks at 1.675 eV (P1), 1.806 eV (P2) and 2.005 eV (P3).

Note

\(\alpha(\vec{\epsilon}, E)\) for z-polarization is generally non-zero in the calculation through k.p model. This is because the eigenstates above the conduction band edge can have the component of valence band Bloch functions and vice versa (band-mixing).

Eigenvalues, transition energies, and occupations

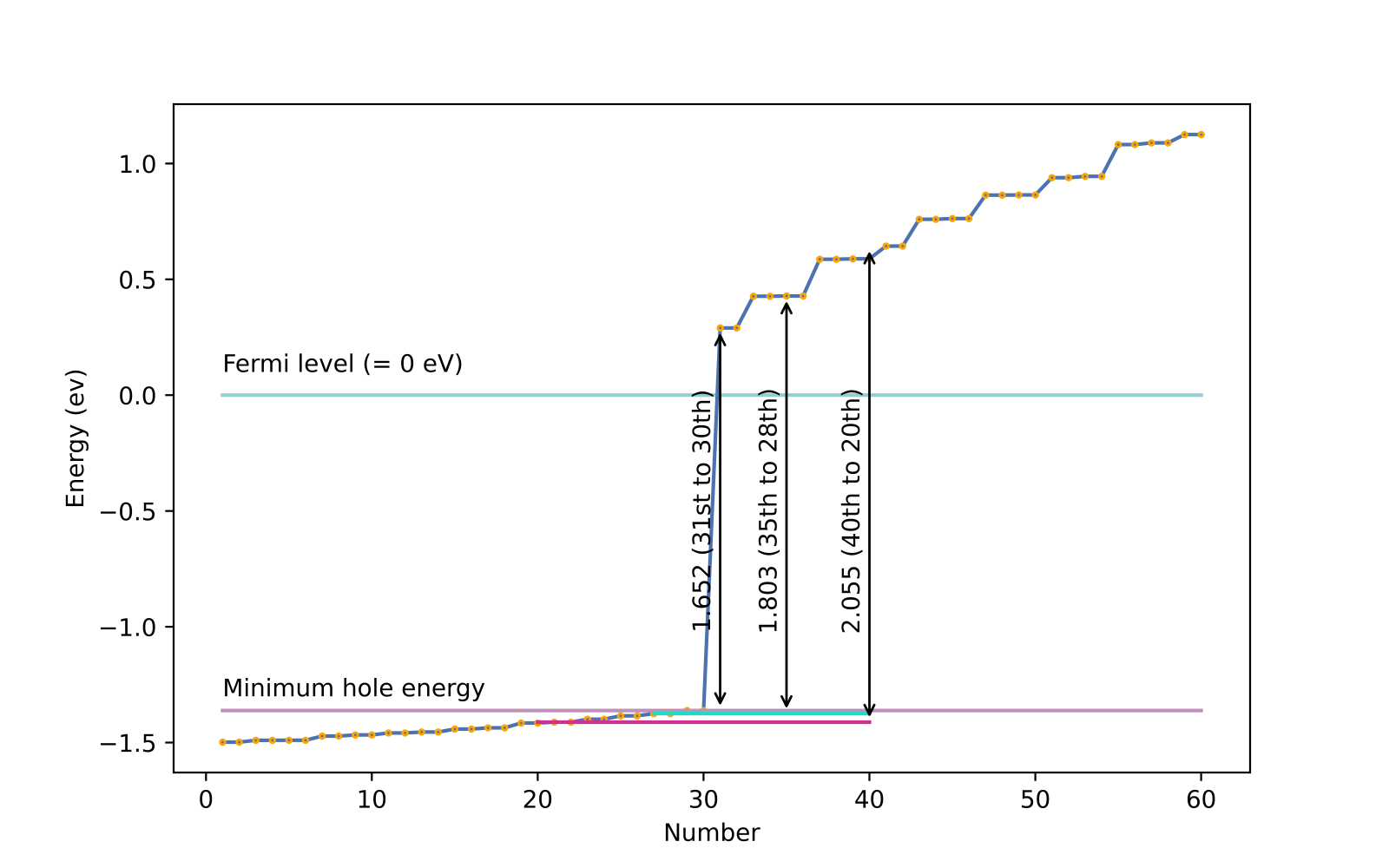
Figure 2.5.12.59 Calculated energy spectrum and the minimum hole energy.

Figure 2.5.12.59 shows the calculated energy eigenvalues at \(\text{k}_z=0\) specified in \Quantum\energy_spectrum_~.dat.

Please note that the output in Quantum\ counts the eigenstates with different spins individually when k.p model is used, while they are counted jointly in Optics\.

The valence band states lie below the Fermi level (0 eV). The minimum hole energy is indicated in Figure 2.5.12.59 with the purple line. It can be seen through a comparison with Figure 2.5.12.58 that the peak in absorption spectrum at P1 corresponds to the transition energy from the minimum hole energy level to the first conduction band state (number 31, 32). Similarly, the peak at P2 corresponds to the transition energy between the minimum hole energy state and the second conduction band state (number 33-36).

The occupation probabilities for each state can be checked from \Optics\occupation_disp_~.datas a function of the 1D Bloch wave vector \(\text{k}_z\):

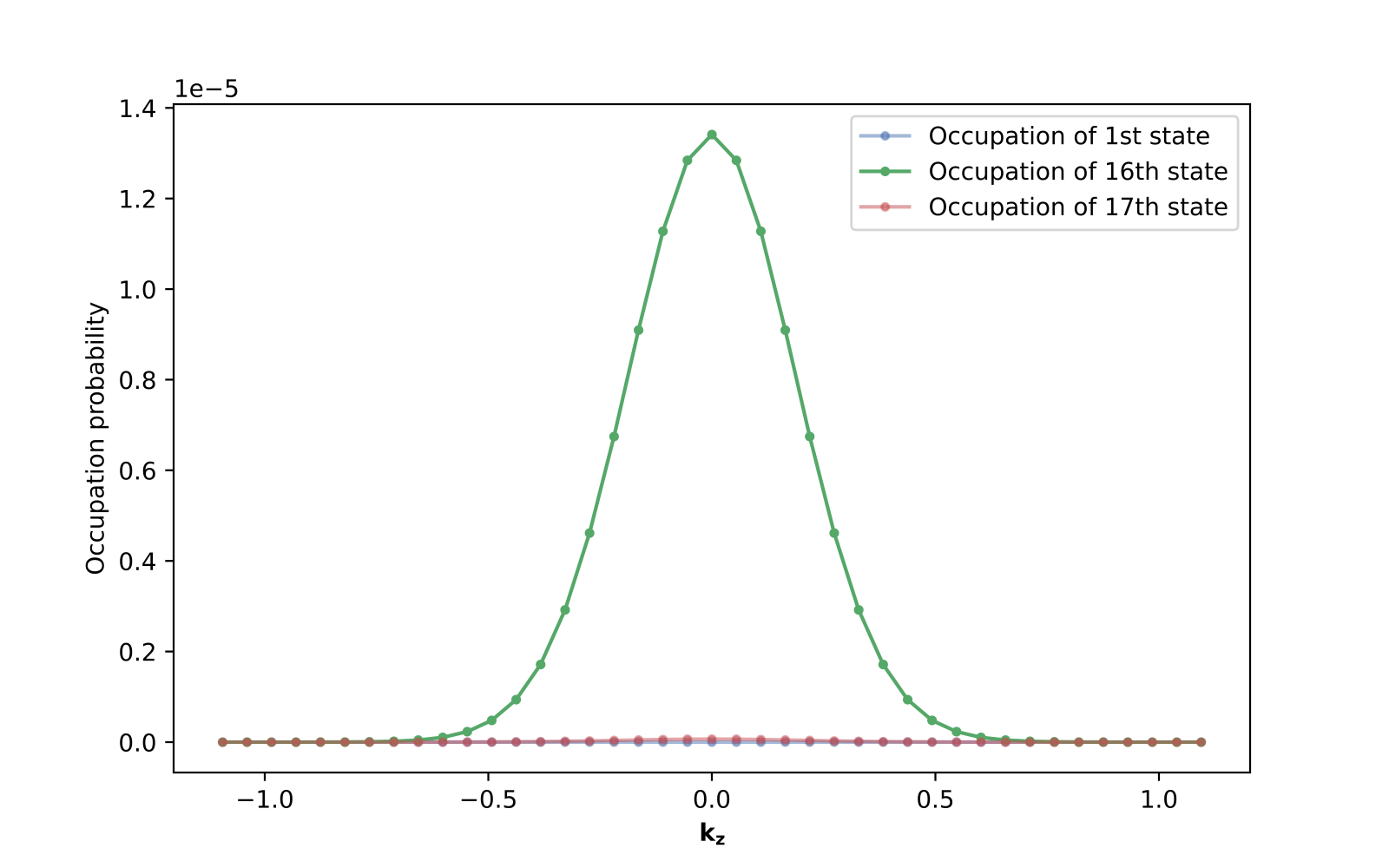
Figure 2.5.12.60 Calculated occupation probabilities for the ground state and 16th excited state as a function of \(\text{k}_z\).

In the above figure, the occupation probabilities are plotted for the 1st and the 16th excited state. The 16th excited state corresponds to the lowest conduction band level.

Note

The eigenstates with different spins are counted individually in Quantum\ when k.p model is used, while they are counted jointly in Optics\.

For example, the two ground states in the conduction band counted as no.31 and 32 in Figure 2.5.12.59 due to spin are put together as one eigenstate in Optics\. Thus \Optics\occupation_disp_~_kp8_16.dat shows the occupation of the ground state in the conduction band and \Optics\occupation_disp_~_kp8_2.dat and \Optics\occupation_disp_~_kp8_17.dat show the 1st excited state in the conduction band (number 33 & 34) in Figure 2.5.12.59.

At \(T=300\text{K},\ k_BT\simeq 0.026\) eV , which is insufficient energy to excite electron carriers to the upper conduction band states.

From the above data of eigenvalues and occupations, we could see which pair of states contributes to each peak in the absorption spectrum Figure 2.5.12.58. In order to understand the magnitude of the peaks and why some pairs of states don’t appear as peaks, we will see the output data for \(|\vec{\epsilon}\cdot\vec{\pi}_{nm}(\text{k}_z)|^2\) next.

Transition intensity (Momentum matrix element)

An important part of the calculation of optical absorption spectra is the transition intensity:

(2.5.12.6)\[T_{nm}(\vec{\epsilon},\text{k}_z)=\frac{2}{m_0}|\vec{\epsilon}\cdot\vec{\pi}_{nm}(\text{k}_z)|^2\]

which has dimensions of energy [eV].

The intensity at \(\text{k}_z=0\) \(\big(T_{nm}(\vec{\epsilon},\text{k}_z=0)\big)\) for each pair of states \((n,m)\) is specified in Optics\transitions_~.txt. These intensities whose “From” states are the ground state are shown here (x-polarization). We can also check the transition energy of each pair of states.

Energy[eV]          From            To              Intensity_k0[eV]                1/Radiative_Rate[s]
2.00196                   10                19                  5.9913                      1.74672e-09
2.00394                   10                20                  1.79227                         5.83325e-09
1.67437                   13                16                  19.9021                         6.2871e-10
1.80179                   14                17                  6.25494                         1.85897e-09

Above are the transitions of interest. The other transitions are ommitted for brevity. The “From” and “To” states tell us which band the transition belongs to. Using this information, we can identify which peaks (P1, P2, P3) correspond to transitions between which bands. This is marked in Figure 2.5.12.59.

There are also the output files that specify the k-dispersion of the transition intensities for each light polarization in Optics\transition_disp_~.dat.

Eigenstates

The probability distributions of the eigenfunctions \(|\psi(\textbf{r})|^2\) can be found in Quantum\probabilities_~.vtr. The amplitude of the envelope function on each Bloch function \(|S\rangle,|X\rangle,|Y\rangle,|Z\rangle\) can be found in Quantum\amplitudes_~_SXYZ.vtr.

The analytcal expression of the eigenfunctions for the cylindrical quantum wire is shown as eq. (2.5.7.1) in this tutorial: Electron wave functions in a cylindrical well (2D Quantum Corral). According to this analytical solution, the eigenfunction has 2 quantum numbers: \(n\) for radial direction and \(l\) for circumferential direction.

Here the amplitudes of eigenfunctions calculated by single-band model are shown. We can see the optical transition from ground state (\(n=1,l=0\)) occurs only to the states with \(l=\pm 1\). The file used for this plot is amplitudes_quantum_region_Gamma_00000.vtr in the single band calculation.

Figure 2.5.12.61 Wave function of the ground state. \((n,l)=(1,0)\)

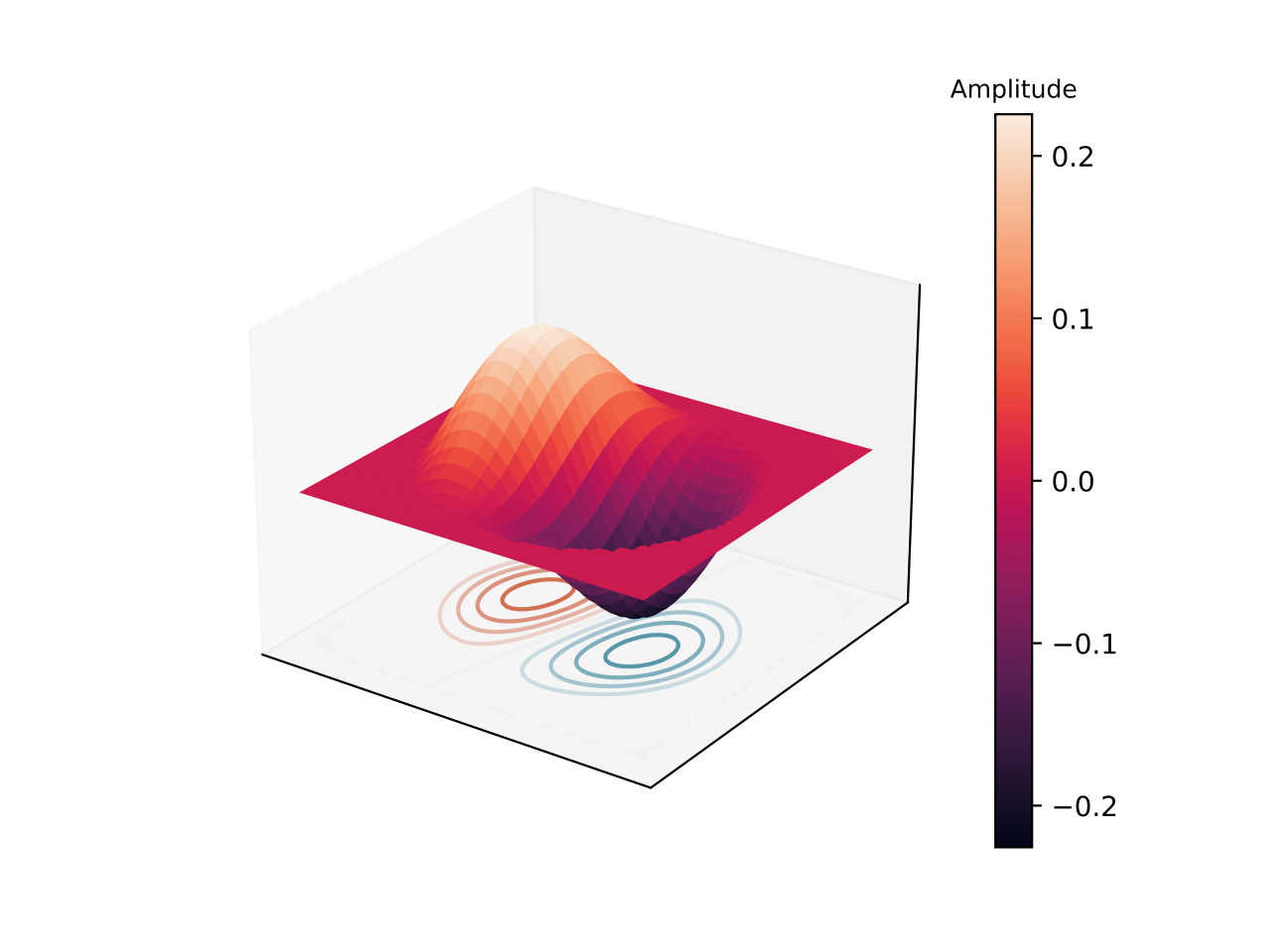
Figure 2.5.12.62 Wave function of the first excited state. \((n,l)=(1,\pm1)\)

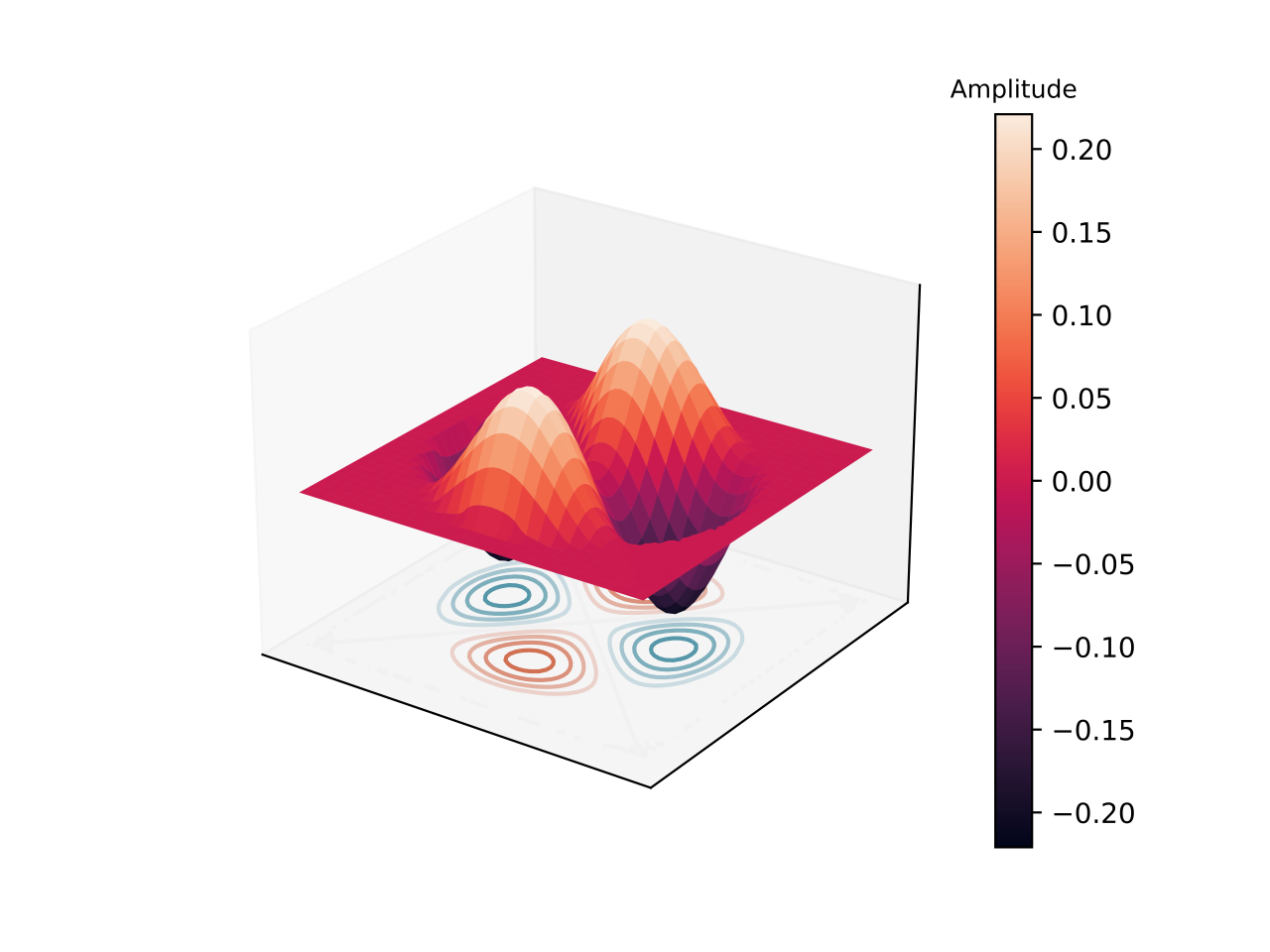
Figure 2.5.12.63 Wave function of the second excited state. \((n,l)=(1,\pm2)\)

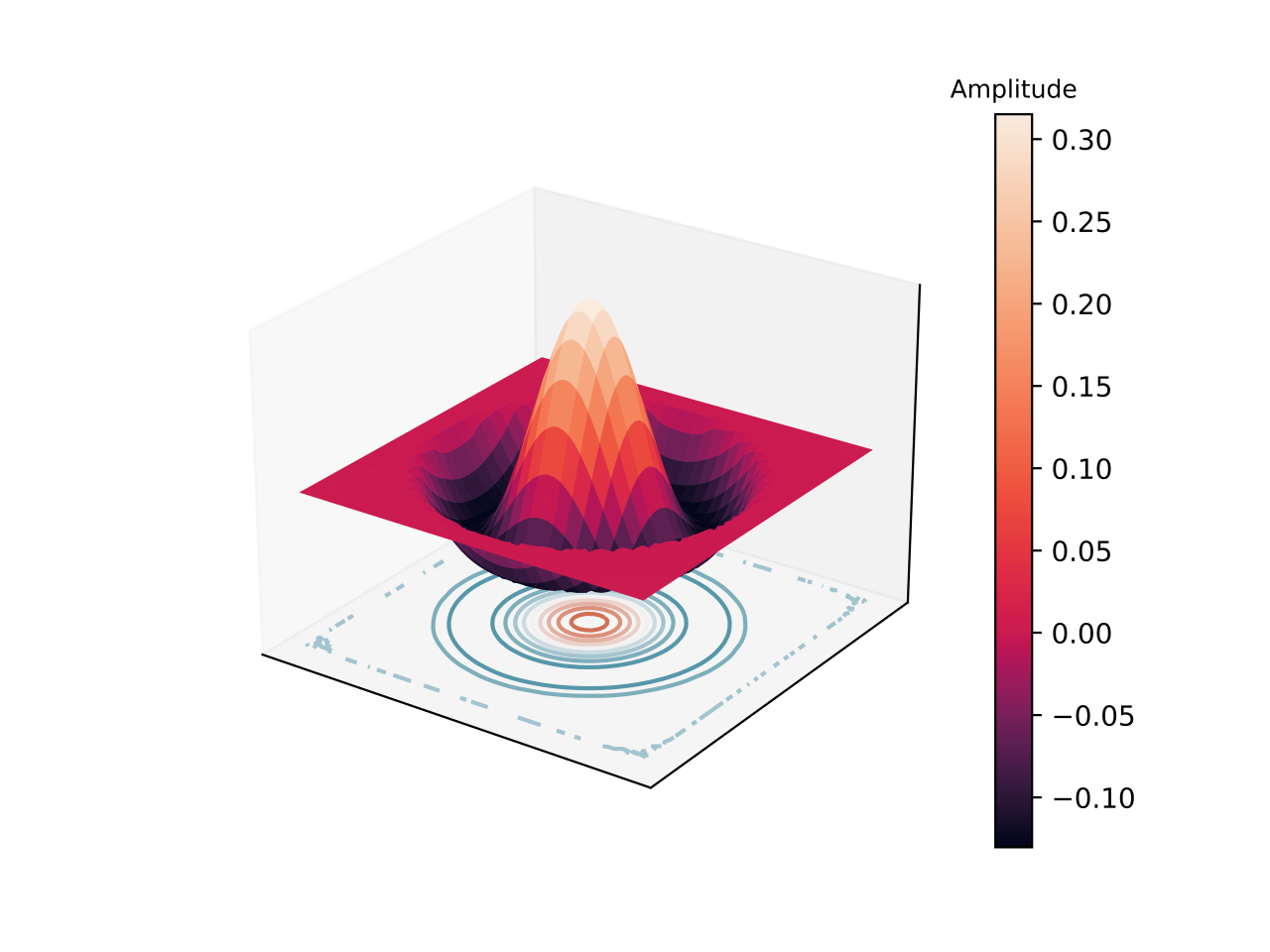
Figure 2.5.12.64 Wave function of the third excited state. \((n,l)=(2, 0)\)

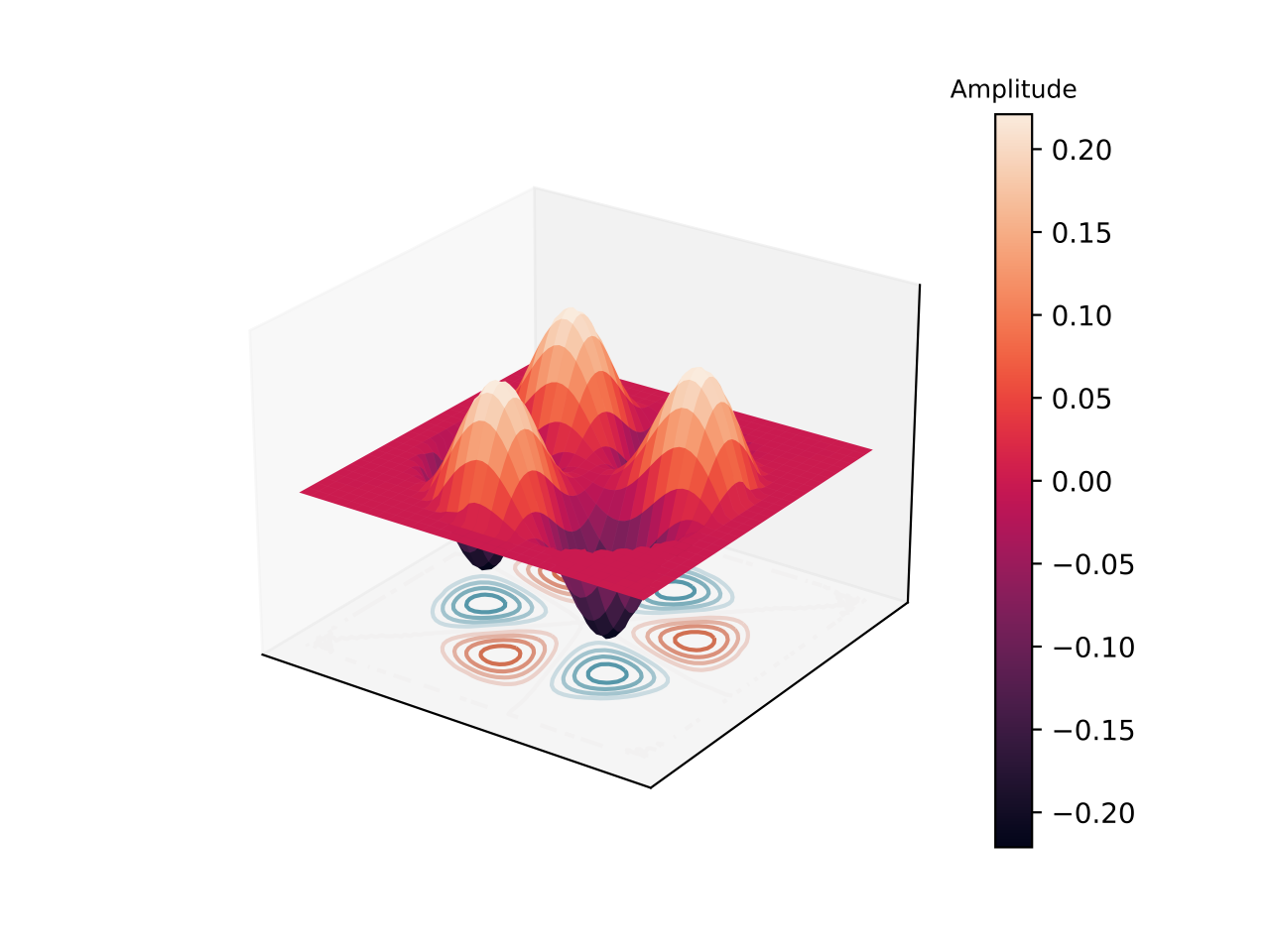
Figure 2.5.12.65 Wave function of the fourth excited state. \((n,l)=(1,\pm3)\)

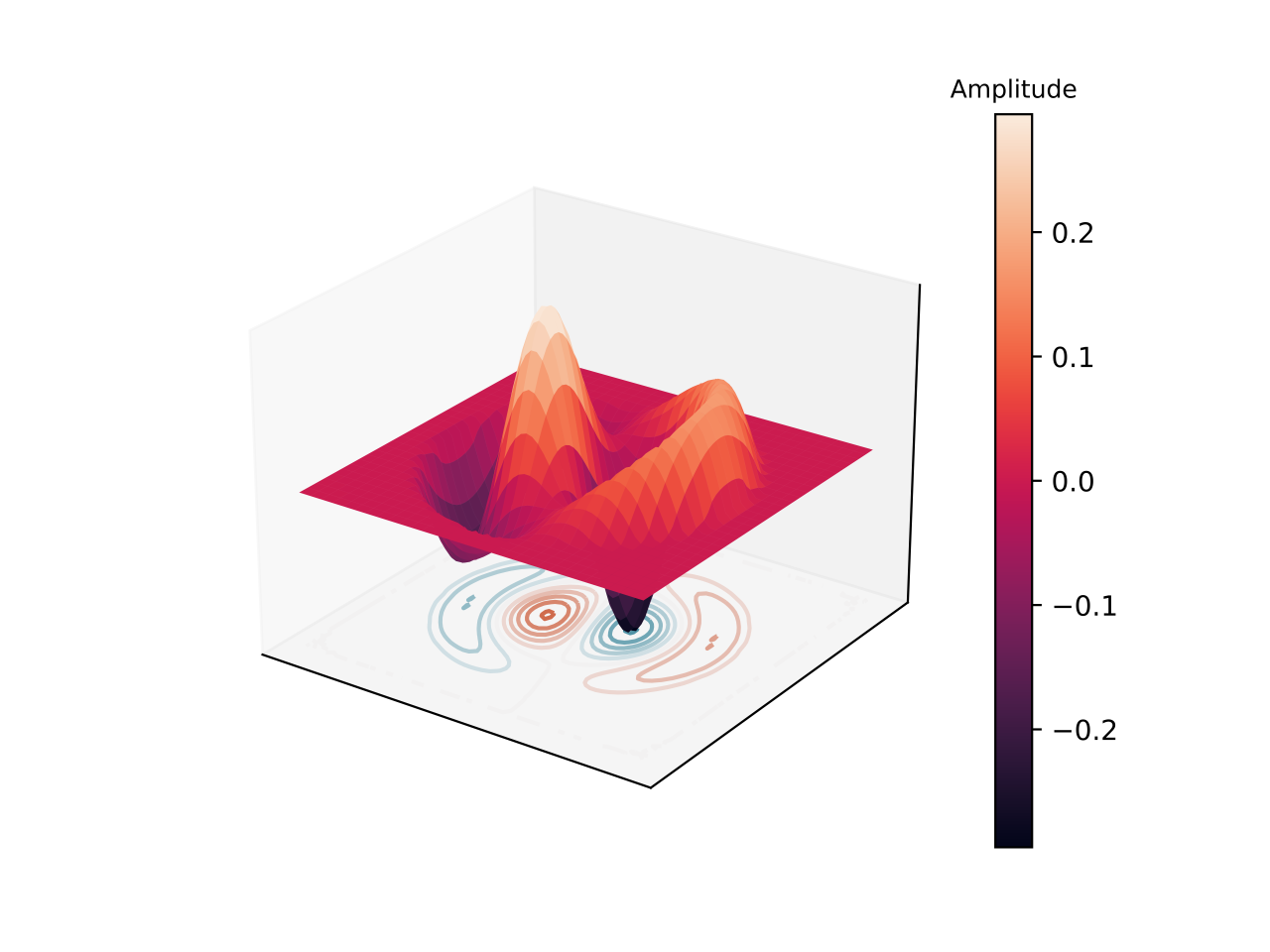
Figure 2.5.12.66 Wave function of the fifth excited state. \((n,l)=(2,\pm1)\)

Wave functions of the energy eigenstates calculated by the single-band model.

Last update: nn/nn/nnnn